
HE-A Steel Beams - Properties of HE-A profiled steel beams.Euler's Column Formula - Buckling of columns.Center Mass - Calculate position of center mass.Cantilever Beams - Moments and Deflections - Maximum reaction force, deflection and moment - single and uniform loads.British Universal Columns and Beams - Properties of British Universal Steel Columns and Beams.Beams - Supported at Both Ends - Continuous and Point Loads - Supporting loads, stress and deflections.Beams - Fixed at One End and Supported at the Other - Continuous and Point Loads - Supporting loads, moments and deflections.Beams - Fixed at Both Ends - Continuous and Point Loads - Stress, deflections and supporting loads.Area Moment of Inertia Converter - Convert between Area Moment of Inertia units.Area Moment of Inertia - Typical Cross Sections II - Area Moment of Inertia, Moment of Inertia for an Area or Second Moment of Area for typical cross section profiles.American Wide Flange Beams - American Wide Flange Beams ASTM A6 in metric units.American Standard Steel C Channels - Dimensions and static parameters of American Standard Steel C Channels.American Standard Beams - S Beam - American Standard Beams ASTM A6 - Imperial units.Beams and Columns - Deflection and stress, moment of inertia, section modulus and technical information of beams and columns.Mechanics - Forces, acceleration, displacement, vectors, motion, momentum, energy of objects and more.the "Section Modulus" is defined as W = I / y, where I is Area Moment of Inertia and y is the distance from the neutral axis to any given fiber." Moment of Inertia" is a measure of an object's resistance to change in rotation direction." Polar Moment of Inertia" as a measure of a beam's ability to resist torsion - which is required to calculate the twist of a beam subjected to torque." Area Moment of Inertia" is a property of shape that is used to predict deflection, bending and stress in beams.I x = (1 / 3) (B y b 3 - B 1 h b 3 + b y t 3 - b1 h t 3) (9)Īrea Moment of Inertia vs. I y = (a 3 h / 12) + (b 3 / 12) (H - h) (8b) Nonsymmetrical ShapeĪrea Moment of Inertia for a non symmetrical shaped section can be calculated as I x = (b h / 12) (h 2 cos 2 a + b 2 sin 2 a) (7) Symmetrical ShapeĪrea Moment of Inertia for a symmetrical shaped section can be calculated as

Rectangular section and Area of Moment on line through Center of Gravity can be calculated as Rectangular Section - Area Moments on any line through Center of Gravity The diagonal Area Moments of Inertia for a square section can be calculated as I y = π (d o 4 - d i 4) / 64 (5b) Square Section - Diagonal Moments The Area Moment of Inertia for a hollow cylindrical section can be calculated as = π d 4 / 64 (4b) Hollow Cylindrical Cross Section The Area Moment of Inertia for a solid cylindrical section can be calculated as I y = b 3 h / 12 (3b) Solid Circular Cross Section The Area Moment of Ineria for a rectangular section can be calculated as I y = a 4 / 12 (2b) Solid Rectangular Cross Section The Area Moment of Inertia for a solid square section can be calculated as Area Moment of Inertia for typical Cross Sections II.
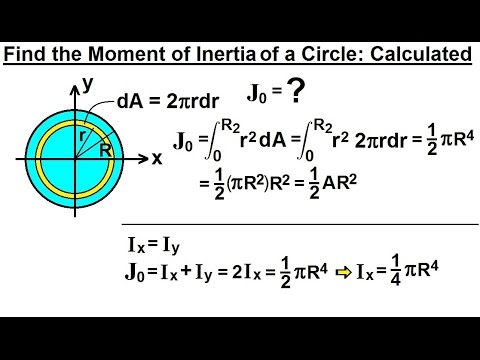
X = the perpendicular distance from axis y to the element dA (m, mm, inches) Area Moment of Inertia for typical Cross Sections I I y = Area Moment of Inertia related to the y axis ( m 4, mm 4, inches 4) The Moment of Inertia for bending around the y axis can be expressed as Y = the perpendicular distance from axis x to the element dA (m, mm, inches )ĭA = an elemental area ( m 2, mm 2, inches 2) I x = Area Moment of Inertia related to the x axis ( m 4, mm 4, inches 4) (9240 cm 4) 10 4 = 9.24 10 7 mm 4 Area Moment of Inertia (Moment of Inertia for an Area or Second Moment of Area)įor bending around the x axis can be expressed as Area Moment of Inertia - Imperial unitsĮxample - Convert between Area Moment of Inertia Unitsĩ240 cm 4 can be converted to mm 4 by multiplying with 10 4
Area Moment of Inertia or Moment of Inertia for an Area - also known as Second Moment of Area - I, is a property of shape that is used to predict deflection, bending and stress in beams.


 0 kommentar(er)
0 kommentar(er)
